Biophysics Textbook Questions - Chapter 7
\(\mathrm{Car \rightarrow + x}\)
\(\mathrm{a_x = 0 \\ \therefore \;x = x_0 + v_0 t}\)
\(\mathrm{Choose \; x_0 = 0 \\ \therefore\; x = v_0t \\ = (28\;m/s)(0.30\;s) \\ = 8.4\;m}\)
\(\mathrm{\therefore \; the \; displacement \; is\; 8.4\; m \; (forward)}\)
Determine speed of slower runner:
\(\mathrm{v_{slow}= \frac{100\;m}{13.0\;s}= 7.69_2\;m/s}\)
Time for faster runner to run \(\mathrm{100 \;m}\) is \(\mathrm{10.0\;s.}\)
In this time, the slower runner has run
\(\mathrm{7.69_2 \frac{m}{s} \times 10.0\;s = 76.9\;m}\)
\(\mathrm{\therefore\; distance\; between \; runners\;is: \\ (100-76.9)m \\ = 23\;m}\)
\(\mathrm{\uparrow + y}\)
\(\mathrm{v_y = v_{oy}+a_y t \\ Assume\; v_{oy}= 0}\)
\(\mathrm{\therefore\; t = \frac{v_y}{a_y}\\ = \frac{900\;m/s}{50.0\; m/s^2}\\ = 18.0\; s}\)
\(\mathrm{x = x_0 + v_0+t+\frac{1}{2}\;a\;t^2}\)
\(\mathrm{Choose\; x_0 = 0}\)
\(\mathrm{\therefore\;x = (2.00\;m/s)(10.0\;s)+ \frac{1}{2}(3.00\;m/s^2)(10.0\;s)^2 \\ = \;170\;m}\)
\(\mathrm{x = x_0+v_0t+\frac{1}{2}at^2}\)
\(\mathrm{Choose\; x_0 = 0}\)
\(\mathrm{\therefore\;x = v_0t+\frac{1}{2}at^2}\)
\(\mathrm{= (15.0\;m/s)(600\;s)+\frac{1}{2}(0.0200\;m/s^2)(600\;s)^2 \\ = 1.26 \times 10^4 m \\ = 12.6\;km}\)
(a)
\(\mathrm{80.0\frac{km}{h} \times \frac{1000\;m}{1\;km}\times \frac{1\;h}{3600\;s}= 22.2\;m/s \\ = 22.2_2\;m/s}\)
(b)
\(\mathrm{Car \rightarrow +x \\ v_0 = 22.2_2\; m/s \\ v = 0\;m/s\\ t = 4.50\;s}\)
\(\mathrm{v = v_0 +at}\)
\(\mathrm{\therefore\; a - \frac{v-v_0}{t} \\ = \frac{(0 - 22.2_2)}{4.50}\\ = 4.94\; m/s^2}\)
(c)
\(\mathrm{x-x_0 = \biggl(\frac{v_0+v}{2}\bigg)t \\ = \bigg(\frac{[22.2_2+0]m/s}{2}\bigg)(4.50) \\ = 50.0\;m}\)
(a)
\(\mathrm{v_0 = ?\\ v^2 = v_0{^2}+ 2a(x-x_0)\\ \therefore\; v_0 = \sqrt{v^2 - 2a (x-x_0)}\\ = \sqrt{0^2 - 2(-0.0490)(25.0)}\\ = 1.57\;m/s \quad (1.56_5\;m/s)}\)
(b)
\(\mathrm{t = ?\\ v= v_0+at\\ \therefore\; t = \frac{v-v_0}{a} \\ = \frac{-1.56_5\;m/s}{-0.0490 \;m/s^2}\\ = 31.9\;s}\)
A high jumper jumps 1.2 m straight up. With what speed did he leave the ground?
This is a straightforward problem in kinematics.
You should be able to make a list of three known variables and one unknown.
Make this list. Be careful with the sign of each quantity.
known variables:
\(\mathrm{s = 1.2\;m \\ v = 0 \\ a = -9.8\;m/s^2}\)
unknown variables: \(\mathrm{u = \;?}\)
Now use the proper equation to solve for \(\mathrm{u.}\)
\(\mathrm{v^2 = u^2 + 2\;a\;s \\ u = \sqrt{v^2 - 2\;a\;s} \\ = \sqrt{0^2-2(-9.8)(1.2)}\\ = 4.9\frac{m}{s}}\)
(a) At top, \(\mathrm{v = 0}\)
\(\mathrm{y = \;?}\)
Use \(\mathrm{v^2 = v_0{^2}+2a(y-y_0)}\)
\(\mathrm{ \therefore\; o^2 = (7.00)^2 +2(-9.80)(y-0)\\ \therefore\; y = 2.50 \;m}\)
(b) Use \(\mathrm{v = v_0+a\;t}\)
\(\mathrm{ \therefore\;o = 7.00 - 9.80 t \\ \therefore\; t = 0.714\;s \quad (0.714_3\;s)}\)
(c) By symmetry, \(\mathrm{t_{up}= t_{down}}\)
\(\mathrm{\therefore\; total t = 2 (0.714_3\;s)= 1.43\;s}\)
OR
Use \(\mathrm{ y = y_0 +v_0t+\frac{1}{2} a\;t^2}\)
At hand, \(\mathrm{y= 0}\)
\(\mathrm{\therefore\; 0 = 0.7.00\;t +\frac{1}{2}(-9.80)t^2 \\ \therefore\; 0 = t(7.00 - 4.90\;t) \\ \therefore\; t = 0\; (initial), \; or\; t = \frac{7.00}{4.90}= 1.43\;s}\)
(d) At hand, \(\mathrm{y= 0}\)
Use \(\mathrm{v_2 = v_0{^2}+2a(y-y_0)}\)
\(\mathrm{ \therefore\; v^2 = (7.00)^2+2(-9.80)(0-0)\\ \therefore\; v = \pm 7.00 \;m/s \rightarrow choose \; v = -7.00\;m/s (down)}\)
A butterfly is flying in a garden. First it travels due south for 6.0 m and then 37 W of N for 10 m. Assuming that the positive x-direction is east, and the positive y-direction is north,
(a) what are the coordinates of the butterfly's final position relative to its initial position?
(b) what are the magnitude and direction of the displacement of the butterfly (relative to its initial position)?
Start by making a diagram, approximately to scale, showing the butterfly's path. Mark on this the vector required in (b).
Calculate the north and west components of the second portion of the flight.
Combine these with the displacement during the first part to find the final coordinates of the flight.
Finally, use the initial coordinates (\(\mathrm{0,0}\)) and the final to find the net displacement and the angle \(\mathrm{\theta.}\)
For the second portion of the flight, the westerly displacement will be:
\(\mathrm{10.0\;m \times \cos(53^\circ) = -6.0\;m}\)
And the northerly component will be:
\(\mathrm{10.0\;m \times \sin(53^ \circ) = 8.0\;m}\)
Therefore the net north-south displacement will be:
\(\mathrm{-6.0\;m + 8.0\;m = + 2.0\;m}\) (ie: It will be 2m north of its initial positon.)
And the east-west displacement will be: \(\mathrm{-6.0\;m}\)
So the final coordinates (in \(\mathrm{m}\)) will be: (\(\mathrm{-6.0, 2.0}\))
The displacement from the starting point (0,0) will be:
\(\mathrm{displacement = \sqrt{(6.0^2 + 2.0^2})\\ =6.3\;m\\ And\; \tan (\theta) = 2/6\\ = 0.333 \\ therefore\; \theta = 18^\circ \; north\;of \; west}\)
\(\mathrm{v_{oz}= +6.60\;m/s\\ v_{ov}= 0\\ a_z = 0 \\ a_y = -2.20\;m/s^2 \\ t = 2.50\; s \\ v_x = v_{ox}+a_xt = (6.60+0)m/s = 6.60\;m/s \\ v_y = v_{oy}+ a_y t = [0+(-2.20)(2.50)]m/s \\ = -5.50\;m/s}\)
\(\mathrm{v = \sqrt{(6.60)^2(5.50)^2}\; m/s\\ = 8.59\;m/s \\ 0 = \tan^{-1} \big(\frac{5.50}{6.60}\big)= 39.8^\circ \\ \therefore \overrightarrow v = 8.59\;m/s\; at\;39.8^\circ \; south \; of \; east}\)
(a)
\(\mathrm{v_{ox}= 3.00\;m/s \\ v_{oy}= 0 \\ a_x = 0\; \therefore v_x = v_{ox}= 3.00\;m/s \\ a_y = -9.80\;m/s^2 \\ y_o = 0\;m \\ y = -5.00\;m}\)
Use \(\mathrm{v_y{^2} = v_{oy}{^2}+2ay (y-y_o)}\)
\(\mathrm{= 0^2+2(-9.80)(-5.00) \\ \therefore v_y = \pm 9.89_9\;m/s}\)
Since \(\mathrm{v_y }\) is down, \(\mathrm{v_y = -9.89_9\; m/s}\)
\(\mathrm{v = \sqrt{(3.00)^2+(9.89_9)^2}\;m/s \\ = 10.3\; m/s}\)
(b)
\(\mathrm{\theta = \tan^{-1}\bigg(\frac{9.89_9}{3.00}\bigg)= 73.1^\circ}\)
\(\mathrm{v_{ox}= (20.0\;m/s) \cos 30.0^\circ = 17.3_2\; m/s \\ v_{oy}= (20.0\;m/s)\sin30.0^\circ = 10.0\; m/s \\ a_y = -9.80\;m/s^2 \\ a_x = 0 \therefore v_x = v_{ox}}\)
First, calculate time in air.
Use:
\(\mathrm{y = y_o+v_{oy}t+\frac{1}{2}a_y\;t^2 \\ 0=0+10.0 t - 4.90\; t^2 \\ \therefore t = 0\; (throwing) \;or\; t = \frac{10.0}{4.90}\; (catching)\\ = 2.04_1\; s}\)
In \(\mathrm{x}\) - direction, \(\mathrm{v_x = const. = v_{ox}}\)
\(\mathrm{\therefore x = x_o+v_{ox}t \\ = 0+(17.3_2\;m/s)(2.04_1\;s)\\ = 35.4\;m}\)
(or \(\mathrm{35.3\;m}\) if more than 4 digits are kept in intermediate answers.)
First find time in the air:
\(\mathrm{y = y_o +v_{oy}t +\frac{1}{2}a_yt^2\\ -3.00 = 0+0 - 4.90 \; t^2 \\ \therefore t = 0.782_5\;s}\)
Then,
\(\mathrm{v_y = v_{oy}+a_yt\\ = 0 - (9.80\;m/s^2)(0.782_5\;s)\\ = -7.66_9\;m/s}\)
To get \(\mathrm{v_x,}\) use:
\(\mathrm{x = x_o +v_{ox}t+\frac{1}{2}a_x t^2 \\ where: x_o = 0\; and\; a_x \; is \; a_x = 0\; \therefore v_x = v_{ox} \\ \therefore\; v_x = v_{ox} = \frac{x}{t}= \frac{4.00\;m}{0.782_53}= 5.11_2\; m/s}\)
\(\mathrm{v = \sqrt{(5.11_2)^2(7.66_9)^2}\;m/s \\ = 9.22\;m/s\\ \theta = \tan^{-1} \bigg(\frac{7.66_9}{5.11_2}\bigg)= 56.3^\circ}\)
\(\mathrm{v_{ox}= 22.0 \cos \theta \\ v_{oy} = 22.0 \sin \theta}\)
In y-direction:
\(\mathrm{y = y_o +v_{oy}t+\frac{1}{2}a_y t^2 \\ \therefore 0=0 +(22.0\sin \theta)t - 4.90 \;t^2 \\ \therefore 22.0 \sin \theta = 4.9 \; t \quad [1]}\)
In x-direction:
\(\mathrm{x = x_o+v_{ox}t\\ \therefore 31.0 = 0 +(22.0 \cos \theta) t\\ \therefore t = \frac{31.0}{22.0 \cos \theta} \cdot Subst.in\quad[1]}\)
\(\mathrm{\therefore 22.0 \sin \theta = \frac {4.9(31.0)}{22.0\cos\theta}\\ \therefore \sin \theta\cos \theta = \frac{4.9(31.0)}{(22.0)^2}= 0.313_q}\)
But \(\mathrm{\sin \theta \cos\theta = \frac{1}{2}\sin 2\theta}\)
\(\mathrm{(\sin 2\theta = \sin (\theta +\theta) = \sin \theta\cos \theta+\cos \theta\sin\theta= 2\sin\theta\cos\theta)}\)
\(\mathrm{\therefore \sin 2\theta = 2(0.313_q)= 0.6276 \\ \therefore 2\theta = \sin ^{-1}(0.6276)= 38.87^\circ \\ \therefore \theta = 19.4^\circ}\)
A bird is flying due north with a speed of \(\mathrm{15\; m\; s.}\) A train is travelling due east with a speed of \(\mathrm{15\; m\; s.}\) What are the magnitude and direction of the velocity of the bird from the point of view of a passenger in a train?
To determine the velocity of the bird relative to a person on the train, we must put ourselves in the frame of reference of the person on the train. To do this, we'll do two quick experiments.
We'll start with the bird remaining stationary. Imagine that you are somewhere on the train, looking at the bird.
From your frame of reference on the train, in which direction did the bird appear to move?
From your frame of reference, the bird appeared to move in a westerly direction at \(\mathrm{15 \;m/s.}\)
Let's allow the bird to fly now to see the other component of its motion.
Now both object are about to move. Again, imagine that you are somewhere on the train, looking at the bird.
The new component is simply the bird's northerly flight at \(\mathrm{15\; m/s.}\)
Now that we have the magnitude and direction of the two components of the bird's movement relative to a person on the train, all that we need to do is make a velocity vector triangle to calculate the net velocity of the bird. Do this on a piece of paper.
And the calculations are:
\(\mathrm{net\; velocity = \sqrt{15^2+15^2}\; m/s\\ = 21.2\; m/s \\ \tan \theta = \frac{15\;m/s}{15\;m/s}= 1.0\\ \theta = 45.0^\circ}\)
In terms of compass directions, the bird's velocity with respect to a person on the train is \(\mathrm{21.2\; m/s}\) to the northwest.
A bird is migrating non-stop a distance of 2000 km. Its destination is due south of its present position. In still air, the bird can fly with a speed of 40 km hr. However, there is a wind of 25 km hr from the west.
(a) In order to make the trip following a straight line path, in what direction should the bird head?
(b) How long will the trip take?
In this problem, we are dealing with motion in 2 different frames of reference:
- motion with respect to the wind and
- motion with respect to the land.
To illustrate this, we'll do a little experiment.
Pretend that the bird is supended in the air like a balloon, with the wind blowing towards the east at 25 km/hr.
Notice that the bird did not move with respect to the wind.
It did move with respect to the ground.
Let's do the experiment again, this time letting the bird move in a westerly direction at 25 km/hr with respect to the wind. (the wind is still blowing at 25 km/hr to the east)
Notice that this time, the bird's westerly motion is counteracted exactly by the easterly motion of the wind, so that the bird did not move with respect to the ground.
Our goal is to point the bird in such a direction so that it heads due south with respect to the ground; That is, so that it has no east-west motion with respect to the ground. Let's review what we know already:
The bird can fly at 40 km/hr with respect to the wind.
In order for the bird to have no east-west motion wrt the ground, it must fly at 25 km/hr to the west wrt the wind.
Thus, in order for the bird to have a net velocity due south with respect to the ground, roughly in what direction should it fly?
In order to have a net velocity due south with respect to the ground, the bird must fly to the south-west in such a drection that its east-west component of motion is 25 km/hr to the west.
The angle can be determined by constructing a velocity vector diagram.
Calculations
\(\mathrm{velocity\; due \; south = \sqrt{40^2 - 25^2}\; km/hr \\ = 31.2\; km/hr}\)
\(\mathrm{\sin \theta = 25/40 = 0.635 \\ \theta = 38.7^\circ}\)
Therefore, the answer to part (a) is that the bird must fly in a direction \(\mathrm{38.7^\circ}\) west of south.
To solve part (b), we simply need to divide the length of the bird's trip by the due south component of its velocity:
\(\mathrm{time = 2000 \;km / 31.2\; km/hr \\ = 64.1 \;hr}\)
A boat is going to head due north, but unfortunately the tide is running out (east) with a speed of 11 m s. If the boat can travel with a maximum speed of 15 m s in still water, what is the maximum speed with which it can head due north relative to the shore?
In this problem, we are dealing with motion in 2 different frames of reference:
- motion with respect to the water, and
- motion with respect to the shore.
We will illustrate this with a little experiment.
Let's simulate the out-going tide.
Note the motion of the boat (which is just floating on the water) with respect to the shore and with respect to the water.
Notice that while the boat did not move with respect to the water as the tide went out, it did move with respect to the shore.
Let's try the experiment again, this time with the boat travelling westward (towards the shore) at 11 m/s with respect to the water.
Notice that the boat moved with respect to the water, but did not with respect to the shore because it's westward motion counteracted exactly the motion of the out-going tide.
We want the boat to travel due north with respect to the shore, that is, without any east-west motion with respect to the shore.
Let's review what we already know:
- the maximum speed of the boat is \(\mathrm{15\; m/s}\) with respect to the water.
- in order to have no motion wrt the shore, the boat must have a velocity of \(\mathrm{11\; m/s}\) to the west wrt the water.
Thus, in order to have a maximum net velocity due north wrt the shore, the boat must move at \(\mathrm{15 \;m/s}\) (wrt the water) in a direction towards the north-west such that its east-west component is \(\mathrm{11\; m/s}\) to the west.
Make a diagram of the velocity vector triangle.
Here is the diagram and calculation.
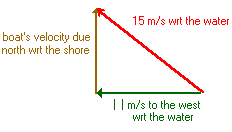
\(\mathrm{velocity\;due\; north = \sqrt {15^2 - 11^2\;m/s }\\=10.2\;m/s}\)
\(\mathrm{v_o = 85.0\frac{km}{h} \times \frac{1\; h}{3600\;s}\times \frac{1000\;m}{1\;km}= 23.61\; m/s \\ v = 0 \\ a = -245\;m/s^2 (note\; that\;- indicates\; slowing \; down) \\ x- x_o = ? \\ v^2 = v_o{^2}+2a\;(x-x_o)\\ \therefore 0^2 = (23.61)^2 + 2(-245)(x - x_o)}\)
Solving for \(\mathrm{(x-x_o)\rightarrow x-x_o = 1.14\;m}\)
As the deer speeds up, the distance travelled is:
\(\mathrm{x_1 = x_o+v_o t+\frac{1}{2} \;a\;t^2}\)
choose \(\mathrm{x_o = 0}\) and \(\mathrm{v_o = 0}\)
\(\mathrm{\therefore x_1 = \frac{1}{2}a\; t^2 \\ = \frac{1}{2}(1.20\;m/s^2)(15.0\;s)^2 \\ = 135\; m\quad [1]}\)
After \(\mathrm{15.0\;s,}\) the velocity is:
\(\mathrm{v = v_o+at \quad where\; v_o = 0 \\ = (1.20\; m/s^2)(15.0\; s)\\ = 18.0\; m/s}\)
Distance travelled at constant \(\mathrm{v}\) is:
\(\mathrm{x_2 = (18.0\;m/s)(30.0\;s)= 540\;m \quad [2]}\)
As tje deer slows down, the distance can be calculated from:
\(\mathrm{v_2 = v_o{^2}+2a\;(x-x_o)\\ 0^2 = (18.0)^2+2(-2.50)(x-x_o)\\ \therefore x-x_o = 64.8\;m\quad [3]}\)
Adding the distances from [1],[2].[3]:
\(\mathrm{(135+540+64.8)\;m = 740\;m}\)
(a) First, find the sidtance travelled by the cheetah while accelerating:
\(\mathrm{x-x_o - \bigg(\frac{v_o+v}{2}\bigg)t\\ = \bigg(\frac{(0+25.0)m/s}{2}\bigg)(5.50\;s)\\ = 68.75\;m}\)
Let \(\mathrm{T = time}\) for cheetah to catch up to gazelle.
In this time, the gazelle travels \(\mathrm{(20.0\;m/s) T.}\)
The cheetah travels \(\mathrm{68.75\;m + (25.0\;m/s)(T-5.50).}\)
These two distances are equal.
\(\mathrm{\therefore\; 20.0\;T = 68.75+25.0(T-5.50)\\ \therefore \; 20.0 \; T = 68.75+ 25.0 \; T - 137.5}\)
Solving for \(\mathrm{T \rightarrow \quad T = 13.8\;s \quad(13.7_5\;s)}\)
(b) The easiest way to find distance travelled is to use the gazelle distance
\(\mathrm{= 20.0\; T\\ = 275\;m }\)
(\(\mathrm{276 \;m}\) if rounded answer for \(\mathrm{T}\) is used)
Let acceleration:
\(\mathrm{time= t_a = 3.80\;s}\)
Then,
\(\mathrm{constant-velocity\; time = t_v = (9.90 - 3.80)s = 6.10\;s }\)
Distance travelled wile accelerating
\(\mathrm{= x_a = x_o+v_o+t_a+\frac{1}{2}a t_a{^2}\\ where \; x_o=0 \; and\; v_o = 0 \\ \therefore x_a = \frac{1}{2} at_a{^2}\quad [1]}\)
At the end of the acceleration phase,
\(\mathrm{v = v_o +at_a = at_a \\ where\; v_o = 0}\)
The runner travels at this \(\mathrm{v}\) for a time \(\mathrm{t_v,}\) and the distance travelled is:
\(\mathrm{x_v = vt_v\\ \therefore x_v = at_at_v \quad [2]}\)
The total distance is 100m.
\(\mathrm{\therefore \;from \; [1]\; \& \; [2],\\ 100 = \frac{1}{2}at_a{^2}+at_at_v \\ = a\big(\frac{1}{2}t_q{^2}+t_at_v\big)\\ \therefore\; 100 = a\big[\frac{1}{2}[3.80]^2+[3.80][6.10]\big] \\ \therefore\; a = 3.29\;m/s^2}\)
(a)
\(\mathrm{+y \uparrow \\ \downarrow a= -g = -9.80\;m/s^2}\)
\(\mathrm{y_o = 0 \rightarrow 0 \\ y = 0\\ t = 4.40\;s}\)
Use \(\mathrm{y = y_o +v_ot+\frac{1}{2}at^2}\)
\(\mathrm{\therefore 0=0 +v_o(4.40)+\frac{1}{2}(-9.80)(4.40)^2}\)
\(\mathrm{Solve\; for\;v_o \Rightarrow v_o = 21.6\;m/s \quad (21.5_6\;m/s)\\ \therefore\; the\; velocity\;was\;21.6\;m/s\; upward.}\)
(b) Use \(\mathrm{v^2 = v_o{^2}+2a\;(y-y_o)}\)
At top, \(\mathrm{v=0.}\)
\(\mathrm{ \therefore 0^2 = (21.56)^2+2(-9.80)(y-0)\\ \Rightarrow\; y = 23.7\;m}\)
\(\mathrm{t_{total}= 4.8\;s \\ v_{sound \;=\; 350\frac{m}{s}}}\)
Plan:
\(\mathrm{t_{total}= t_{rock}+t_{sound}}\)
First:
\(\mathrm{y_{false}= how\;tall\;the\;building\;would\;be\;if\;speed\;of\; sound\;was\;infinite}\)
\(\mathrm{y_{false}= y_0+v_0t_{total} +\frac{1}{2}g(t_{total})^2\\ y_{false}= 0+0_\frac{9.8}{2}(4.8)^2\\ y_{false}= 112.9\;m}\)
\(\mathrm{t_s = how\;long\;it\;actually\;took\;the\;sound\;to\;travel}\)
\(\mathrm{t_s = \frac{y_{false}}{v_s}\\ t_s = \frac{112.9\;m}{350\frac{m}{s}}\\ t_s = 0.323\;s}\)
\(\mathrm{t_{rock} = t_{total}-t_{sound}\\ t_{rock}= 4.800 - 0.323 \\ t_{rock}= 4.48\;s}\)
\(\mathrm{y_{actual}= y_0+v_0t_{rock}+\frac{1}{2}g(t_{rock})^2\\ y_{actual}= \frac{1}{2}9.8(4.48)^2\\ \therefore y_{actual}= 100\;m}\)
(a)
\(\mathrm{\Delta x_a = (855\;m)\cos 25.06^\circ = 774.9\;m \\ \Delta y_a = (855\;m)\sin 25.0^\circ = 361.3\;m}\)
\(\mathrm{\Delta x_b = (560\;m)\sin 22.0^\circ = 209.8\;m \\ \Delta y_b = (560\;m)\cos 22.0^\circ = 519.2 \;m}\)
\(\mathrm{\therefore\; total\; \Delta x = (774.9 + 209.8)\;m = 984.7\;m \\ total\;\Delta y = (361.3+519.2)\;m = 880.5\;m}\)
\(\mathrm{\Delta r\sqrt{(880.5)^2+(984.7)^2}\;m\\ = 1.32\times 10^3\;m \quad(1321\;m)\\ \theta = \tan^{-1}\bigg(\frac{880.5}{984.7}\bigg)= 41.8^\circ\\ \therefore \; \Delta \overrightarrow r= 1.32 \times 10^3 \;m \; at \; 41.8^\circ\; N\; of\; E}\)
(b) \(\mathrm{4.40\;min = 264\;s}\)
\(\mathrm{avg.\;speed = \frac{distance}{\Delta t}= \frac{(855+560)\;m}{264\;s}= 5.36\;m\\ \overrightarrow v_{av}= \frac{\Delta \overrightarrow r}{\Delta t}= \frac{1321\;m, 41.8^\circ \;N\;of\;E}{2645}\\ = 5.00\;m/s, 41.8^\circ\; N\; of\; E}\)
(a)
\(\mathrm{a_x = (0.760)\cos 47.0^\circ = 0.518_3\;m/s^2 \\ a_y = (0.760)\sin 47.0^\circ = 0.555_8\;m/s^2}\)
\(\mathrm{v_x = v_{ox}+ a_x t\\ = 4.20+ (0.518_3)(10.0)\\ = 9.38\;m/s \quad(9.38_3\; m/s)}\)
\(\mathrm{v_y = v_{oy} + a_y t\\ = 3.35+(0.555_8)(10.0)\\ = 8.91\;m/s\quad (8.90_8\;m/s)}\)
(b)
\(\mathrm{v = \sqrt {(8.90_8)^2+(9.38_3)^2}\;m/s \\ = 12.9\;m/s \\ \theta = \tan^{-1}\bigg(\frac{8.90_8}{9.38_3}\bigg)= 43.5^\circ}\)
(a)
\(\mathrm{v_{ox} = 2.10\cos 32.0^\circ = 1.78_1\;m/s\\ v_{oy}= 2.10\sin 32.0^\circ = 1.11_3\;m/s \\ a_y = -9.80\;m/s^2}\)
\(\mathrm{y = y_o+v_{oy}t+\frac{1}{2}a_yt^2\\ \therefore -15.0 = 0+1.11_3t-4.90\;t^2 \\ \therefore 4.90\;t^2 - 1.11_3t-15.0=0 \\ \therefore t=\frac{1.11_3\pm \sqrt{(1.11_3)^2-4(4.90)(-15.0)}}{9.80}\\ -\; need\; t>0 \therefore choose\; positive\; root\\ \Rightarrow t = 1.87\;s\quad (1.86_7\;s)}\)
(b)
\(\mathrm{x = x_o+v_{ox}t\\ = 0+(1.78_1)(1.86_7)\\ = 3.32_5\;m\\ \therefore \Delta r = \sqrt{(15.0)^2+(3.32_5)^2\;}\;m\\ = 15.4\;m}\)
\(\mathrm{v_{ox}= 22.0\cos 46.0^\circ = 15.2_8\;m/s\\ v_{oy}= 22.0 \sin 46.0^\circ = 15.8_3 \;m/s\\ a_y = -9.80\;m/s^2 \\ a_x = 0\\ x = x_o+v_{ox}t\\ \therefore 25.0 = 0 + 15.2_8 t \\ \Rightarrow t = 1.63_6 \;s \\ y = y_o+v_{oy}t+\frac{1}{2}a_y t^2 \\ = 0+(15.8_3)(1.63_6)- (4.90)(1.636)^2 \\ = 12.7_8 \;m }\)
\(\mathrm{\therefore}\) the ball is \(\mathrm{(12.7_8 - 2.90)\;m = 9.9\;m}\) above the crossbar
(a)
\(\mathrm{v_{ox = v_o \cos 14.0^\circ}}\) and \(\mathrm{v_{oy}= v_o \sin 14.0^\circ}\)
\(\mathrm{a_x = 0}\) and \(\mathrm{a_y = -9.80\;m/s^2}\)
In x-direction:
\(\mathrm{x = x_o+v_{xo}t\quad [1] \\ \therefore 240 = 0+v_o(\cos 14.0^\circ)t \quad [2]}\)
In y-direction:
\(\mathrm{y = y_o +v_{oy}t+\frac{1}{2}a_y\;t^2 \\ \therefore 0=0+v_o(\sin 14.0^\circ)t -4.90\;t^2 \\ \therefore t = \frac{v_o \sin 14.0^\circ}{4.90}\quad [3] \quad Subst.in \; [2].\\ \therefore 240 = v_o\cos 14.0^\circ \times \frac{v_o\sin 14.0^\circ}{4.90}\\ \Rightarrow v_o{^2} = 5010\;m^2/s^2 \\ and\; v_o = 70.8\;m/s \quad (70.7_8\;m/s)}\)
(b)
Now, \(\mathrm{v_0 = (70.7_8+0.6)\;m/s = 71.3_8\;m/s}\)
Eq. \(\mathrm{[3]}\) still applies: \(\mathrm{\therefore t = \frac{71.3_8 \sin 14.0^\circ}{4.90}= 3.52_4\;s}\)
Now use \(\mathrm[1]\) :
\(\mathrm{x = x_o + v_{ox}t\\ = 0+(71.3_8 \cos 14.0^\circ)(3.52_4\;s)\\ = 244\;m, i.e., 4\;m \; farther}\)
(c)
Now the angle is \(\mathrm{14.5^\circ}\) and \(\mathrm{v_o = 70.7_8 \;m/s.}\)
Use \(\mathrm[3]\) with angle of \(\mathrm{14.5^\circ}\) :
\(\mathrm{t = \frac{70.7_8 \sin 14.5^\circ}{4.90}= 3.61_7\;m/s}\)
Use \(\mathrm{[1]}\) again:
\(\mathrm{x = 0+(70.7_8 \cos 14.5^\circ)(3.61_7)\\ = 248\;m, i.e., 8\;m\; farther}\)
\(\mathrm{v_{ox}= 39.0 \cos 50.0^\circ = 25.0_7 \;m/s \\ v_{oy}= 39.0 \sin 50.0^\circ = 29.8_8\;m/s\\ a_x = 0\\ a_y = -9.88\;m/s^2}\)
\(\mathrm{y = y_o +v_{oy}t+\frac{1}{2}a_y t^2 \\ \therefore 0 = 0+29.8_8t-4.90\;t^2\\ \therefore t = \frac{29.8_8}{4.90}= 6.09_7 \;s }\)
\(\mathrm{x = x_o +v_{ox}t \\ = 0+(25.0_7)(6.09_7)\\ = 152.9\;m}\)
\(\mathrm{\therefore}\) the fileder must run \(\mathrm{(152.9-125)= 27.9\;m}\) in \(\mathrm{6.09_7\;s}\) to catch the ball
\(\mathrm{\therefore his\; speed\; = \frac{27.9\;m}{6.09_7\;s}= 4.6\;m/s}\)
Let \(\mathrm{B = bird,}\) \(\mathrm{A = air}\), \(\mathrm{G = ground}\)
Choose \(\mathrm{+x \;West,}\) \(\mathrm{+y \;South}\)
\(\mathrm{\overrightarrow v_{BG} = \overrightarrow v_{BA}+\overrightarrow v_{AG}}\)
\(\mathrm{v_{BA, x}= 35.0 \cos 30.0^\circ = 30.3, \;km/h \\ v_{BA, y}= 35.0 \sin 30.0^\circ = 17.50\;km/h\\ v_{AG,x}= 0\\ v_{AG, y}= 67\;km/h \\ \therefore v_{BG, x} = v_{BA,x} + v_{AG, x}= 30.3, \;km/h \\ v_{BG, y}= v_{BA, y}+v_{AG,y}= 84.50\; km/h}\)
\(\mathrm{v_{BG}= \sqrt{(30.3_1)^2+(84.50)^2}\;km/h\\ = 89.7_7\;km/h}\)
\(\mathrm{\therefore}\) distance trevalled in \(\mathrm{1.50\;h}\) is \(\mathrm{(89.7_7\;km/h)(1.50\;h)= 135\;km}\)
\(\mathrm{\theta = \tan^{-1}\bigg( \frac{84.50}{30.31} \bigg)= 70.3^\circ}\)
\(\mathrm{\therefore}\) displacement is \(\mathrm{135\;km}\) , \(\mathrm{70.3^\circ}\) south of west
Let P = plane, A = air, G = ground
Choose \(\mathrm{+x\; East}\) , \(\mathrm{+y\; South.}\)
\(\mathrm{\overrightarrow v_{PG}= \overrightarrow v_{PA} + \overrightarrow v_{AG}}\)
\(\mathrm{v_{PA, x}= 320 \sin 45.0^\circ = 226.3 \;km/h \\ v_{PA, y}= 32.0 \cos 45.0^\circ = 226.3 \;km/h \\ v_{AG, x}= 79.0 \sin 22.0 ^\circ = 29.5_9 \;km/h \\ v_{AG, y}=-79.0 \cos 22.0^\circ = -73.2_5\;km/h}\)
Then,
\(\mathrm{v_{PG, x}= v_{PA,x}+v_{AG, x}= 255.9\;km/h\\ v_{PG,y}= V_{PA,y}+v_{AG,y}= 153.1\;km/h}\)
\(\mathrm{v_{PG}= \sqrt{(255.9)^2+(153.1)^2}\;km/h \\ = 298\;km/h \\ \theta = \tan^{-1} \bigg( \frac{153.1}{255.9}\bigg)=30.9^\circ \\ \therefore \overrightarrow v_{PG}= 298\;km/h, 30.9^\circ \; south\; of\; east}\)