Study Guide 7 - Ionizing Radiation
- \(\mathrm {? =\; ^{12} C+ \beta^- + v}\)
Rewrite this equation with the atomic mass number and the atomic number (nuclear charge) explicit for each item in the equation:
\(\mathrm {? =\;\begin {smallmatrix}^{12} \\ _6 \end{smallmatrix} \;C\; +\; \begin {smallmatrix} ^0 \\ _{-1} \end{smallmatrix}e + v }\)The equation must balance so that the total atomic mass number and the total atomic number is the same on each side.
\(12 + 0 + 0 = 12 \\ 6 - 1 + 0 = 5\)
Equation is:\(\mathrm {\begin {smallmatrix} ^{12} \\ _5 \end{smallmatrix} ? =\;\begin {smallmatrix}^{12} \\ _6 \end{smallmatrix} \;C\; +\; \begin {smallmatrix} ^0 \\ _{-1} \end{smallmatrix}e + v }\)
Go to the Periodic Table to determine the unknown.
- \(\mathrm {\begin{smallmatrix} 224 \\ 88 \end{smallmatrix} Ra = ? +\; _2^4 \alpha}\)
\(224 = 220 + 4 \\ 88 = 86 + 2\)
\(\mathrm {\begin{smallmatrix} 224 \\ 88 \end{smallmatrix} Ra = \begin {smallmatrix} 220 \\ 86 \end {smallmatrix} ? +\; _2^4 \alpha}\)Go to the Periodic Table to identify the unknown.
- \(\mathrm {\begin{smallmatrix} 210 \\ 83 \end{smallmatrix} \; Bi = \begin{smallmatrix} 210 \\ 84 \end{smallmatrix} \; Po\; + \;?}\)
\(210 = 210 + 0 \\ 83 = 84 - 1\)
\(\mathrm {\begin{smallmatrix} 210 \\ 83 \end{smallmatrix} \; Bi = \begin{smallmatrix} 210 \\ 84 \end{smallmatrix} \; Po\; + \begin{smallmatrix}0 \\ -1 \end{smallmatrix}\;?}\)
What is this particle?
\(\mathrm {\begin{smallmatrix} 210 \\ 83 \end{smallmatrix} \; Bi = \begin{smallmatrix} 210 \\ 84 \end{smallmatrix} \; Po\; + \begin{smallmatrix}0 \\ -1 \end{smallmatrix}\;e}\)
The unknown is an electron or \(\beta \) particle.
- The physical half life of an isotope is 15 days. What is the corresponding decay constant in day-1 and s-1?
The relation between half life and decay constant is:
\(\mathrm {\lambda = 0.693/T_½}\)
\(\mathrm {For\; T_½ = 15 \; day,\; \lambda = 0.693/15 = 0.0462 \;day^{-1}}\)\(\mathrm {0.0462 \; day-1(1/24 \times 3600) day/s = 5.35 \times 10^{-7} s^{-1}}\)
- The values of \(\mathrm {\lambda_ p}\) and \(\mathrm {\lambda_b}\) for a particular radioactive isotope in humans is 0.080 day-1 and 0.050-1 respectively. What is the effective half life of the isotope in humans?
\(\mathrm {\lambda _e = \lambda_p + \lambda_b = 0.080 + 0.050 = 0.130\; day^{-1}}\)
\(\mathrm {T_½ = 0.693/\lambda_e = 0.693/0.130 = 5.3 \;days}\)
- Two goats at Port Hopeless, Ontario accidentally get into a field containing radioactive grass. The goat "Aristophanes" eats twice as much grass as the goat "Demetrius". However Aristophanes' metabolism is 1.5 times faster than that of Demetrius and the ingested isotope has in this case a biological decay constant which is directly proportional to metabolism. If the biological decay constant is 0.10 day-1 in Demetrius, how long will it be until the two goats contain the same concentration of isotope? Assume that the physical half life is very long compared to the biological half lives.
\(\mathrm {Since\; _ ½T_p \;is \;very \;long \;then \; \lambda_p = 0}\)
\(\mathrm {Therefore\; \lambda_e = \lambda_b = \lambda}\)\(\mathrm {Also\; \lambda (A) = 1.5 \lambda (D)}\)
For Aristophanes:
\(\mathrm {NA = 2N_0{e^{-\lambda (A) t}} = 2N_0e ^{-1.5\lambda(D)t}}\)For Demetrius:
\(\mathrm {N_D = N_0 = N_0e ^{-\lambda(D)t}}\)When \(\mathrm {N_A = N_D}\)
\(\mathrm {2N_0e^{-1.5l\lambda (D)t} = N_0e^{-\lambda(D)t}}\)Taking natural logarithms:
\(\mathrm {ln \;2 - 1.5 \lambda (D) t = -\lambda (D) t}\)\(\mathrm {0.5 \lambda (D) t = ln \;2 \\ t = ln\; 2/(0.5)(0.10) = 14\; days}\)
- The count rate 300 cm from a radioactive source is 900 C/min. The detector is moved so that the count rate is 600 C/min. How far is the detector from the source? How does the count rate vary with distance?
\(\mathrm {C ~ 1/d^2 \\ C_1/C_2 = (d_2/d_1)^2 \\ d_2\;^2 = (900/600)(300)^2 \\ d_2 = (9/6)^½ 300 = 370 \;cm}\)
- It is discovered experimentally that all the γ s from a particular source are just absorbed by 5.0 mm of copper (density 9.00 gm/cm3). Would 9.0 cm of zircon (density 4.70 gm/cm3) absorb all the γ s from this source?
\(\mathrm {I = I_0e^{-\mu_m \rho x }}\)
Copper: \(\mathrm {\rho x = 9 \times 5 = 45}\)
Zircon: \(\mathrm {\rho x = 4.7 \times 9 = 42.3}\)
Since \(\mathrm {\mu_ m}\) is the same in each case then if \(\mathrm {\rho x = 45}\) just absorbs all the \(\gamma\) s then \(\mathrm {\rho x = 42.3}\) will be insufficient.
- A graph of \(\mathrm {\ell n \; y \; vs\; x}\) is plotted below. What is the equation that relates y to x? Be sure to specify the numerical values and units of all constants in the equation.
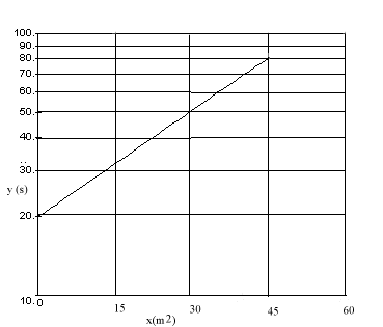
This is a semi-log plot so
\(\mathrm {y = c \;e^{kx} \\ \ell n \;y = \ell n \;c + kx}\)
Therefore a semi-log plot gives a straight line.
In the graph c is the intercept so c = 20 s.
The slope of the graph is k. Using the points (45,80) and (0,20)\(\mathrm {k = \frac{\ell n80 - \ell n20}{45 - 0}= \frac{\ell n (80/20)}{45}= \frac{1.386}{45}= 0.031}\)
Find the units of k:
The quantity kx must be dimensionless (since it appears as an exponent).
Since x has units m2 then k must have units m-2
\(\mathrm {k = 0.031 \;m^{-2}}\)
- A 10.0 gram sample of tissue receives a dose of X-rays of 1.0 sievert. The \(\mathrm {W_R}\) for this particular radiation is 1.10.
(a) What is the dose in grays?
Equivalent Dose (gray) = QF X Dose (sievert)
Dose = 1.0/1.01 = 0.91 gray
(b) How much energy in joules is absorbed by the sample?
\(\mathrm {Energy = 0.91 (gray) \times 1.00 (J/kg) \times 10\times 10^{-3} (kg) \\ = 9.09\times 10^{-3} \;J}\)
- Your dentists asks you if an alpha particle source might give him a better picture of your teeth than his X-ray tube. Would you encourage or discourage this change? Why?
Alpha particles have a high LET (linear energy transfer) so would be entirely stopped by the skin where they might do much damage. They would not reach the teeth.
