Trigonometry 1

The questions are based on the figure below: 

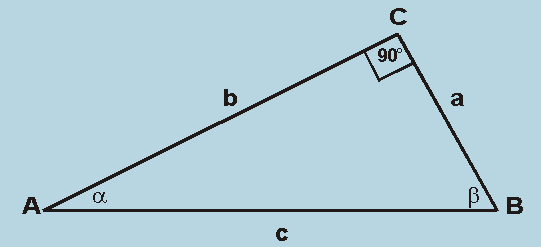
Diagram of right angle triangle with angles A,B,C indicated, sides a, b, c indicated and angles alpha and beta indicated

  1. The area of the triangle is given by ... 

    (A)   \(A = ½ (a+b)c\) 
    (B)   \(A = ½\; a · b\) 
    (C)   \(A = ½\; a · c \)
    (D)   \(A = a^2 + b^2 - c^2\) 

    Correct = B


     
  2. Which expression is wrong: 

    (A)   \(c^2 = a^2 + b^2\) 
    (B)   \(c^2 - a^2 = b^2\)  
    (C)   \(b^2 - c^2 = a^2 \)
    (D)   \(b^2 - a^2 + 2a^2 = c^2\)  

    Correct = C


     
  3. Which expression regarding the values of the angles is right: 

    (A)   \(\alpha + \beta < 90^\circ\) 
    (B)   \(\alpha + \beta > 90^\circ\) 
    (C)   \(180^\circ + (\alpha + \beta) = 360^\circ \)
    (D)   \(180^\circ - \alpha = 90^\circ + \beta\) 

    Correct = D


     
  4. If the length of side \(c\) is \(13\; \text {cm},\) the length of side a is \(5\; \text{cm},\) what will be the length of side \(b\)? 

    (A)   \(7\; \text {cm}\) 
    (B)   \(8\; \text {cm}\) 
    (C)   \(12\; \text {cm}\) 
    (D)   \(18\; \text{cm}\) 

    Correct = C


     
  5. Which expression is correct? 

    (A)   \(\sin \beta = c/b\) 
    (B)   \(\sin \beta = b/a\) 
    (C)   \(\sin \alpha = b/c\) 
    (D)   \(\sin \alpha = a/c\) 

    Correct = D


     
  6. Spot the mistake: 

    (A)   \(\sin \beta = b/a\) 
    (B)   \(\sin \alpha = a/c\) 
    (C)   \(\cos \beta = a/c\) 
    (D)   \(\cos \alpha = b/c\) 

    Correct = A


     
  7. Identify the wrong expression: 

    (A)   \(a = c · \sin \alpha\)  
    (B)   \(a = c · \cos \alpha\)  
    (C)   \(b = c · \sin \beta\)  
    (D)   \(a = c · \cos \beta\)  

    Correct = B


     
  8. The \(\tan\) of angle \(\beta\) is given by ... 

    (A)   \(\tan \beta = b/a\) 
    (B)   \(\tan \beta = a/b\) 
    (C)   \(\tan \beta = b/c\) 
    (D)   \(\tan \beta = a/c\) 

    Correct = A


     
  9. The sin of \(30^\circ \) is ... 

    (A)   1 
    (B)   2/3  
    (C)   1/2 
    (D)   1/4  

    Correct = C


     
  10. The cos of \(60^\circ \) is the same as ... 
    (A)   \(\tan 45^\circ \)  
    (B)   \(\tan 60^\circ\)  
    (C)   \(\sin 30^\circ\) 
    (D)   \(\sin 60^\circ \) 

    Correct = C