Problem 2-99 - v-t graph - Part 6 - (a)
Two cars, \(A\) and \(B\), are stopped for a red light beside each other at an intersection. The light turns green and the cars accelerate. Their velocity time graphs are shown in the figure below. (The positive direction is "forward.")
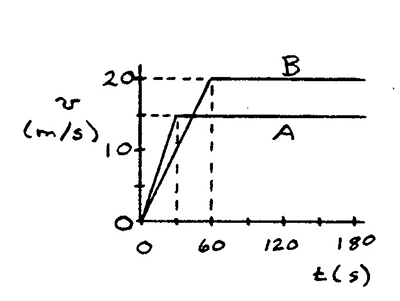
(a) At what time(s) do \(A\) and \(B\) have the same velocity?
(b) When does \(B\) overtake \(A\)? (Hint: Their displacements must be equal at that time and displacement can be found from a velocity time graph.)
(c) How far have the cars traveled when \(B\) overtakes \(A\)?
Accumulated Solution
The velocities are equal at \(t = 45\; s\)
\(d_{A,60} = (1/2)(15\;m/s)(30\;s) + (15\;m/s)(30\;s) = 675\; m\)
\(d_{B,60} = (1/2)(20\;m/s)(60\;s) = 600\; m\)
Correct.
At \(t = 60\; s, A\; \text {is} \;75\; m\) further than \(B\).
After \(60\; s\), both \(A\) and \(B\) are:
(A) accelerating
(B) at rest
(C) moving at constant speed.