Biophysics Problem 22
For the example on the tension in the deltoid muscle of the arm, determine how the expression for the tension is changed if a weight \(W\) is placed in the hand.
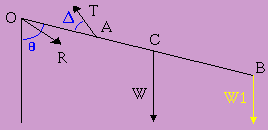
This example is on page 102 of your text. Refer back to the diagram and imagine an additional force W acting vertically downwards at \(B.\)
In the text example, \(R\) passes through \(O\) and exerts no moment about it. The first equation in the example indicates that the torques of \(T\) and \(W\) about \(O\) sum algebraically to zero.
We have:
\((OA) \sin \Delta\) is the perpendicular distance from \(O\) to the line of action \(T\)
\((OC) \sin \theta\) is the perpendicular distance from \(O\) to the line of action \(W\)
And from the new force:
\((OB) \sin \theta\) is the perpendicular distance from \(O\) to the line of action of the \(\text{New Force}\; W_1\)
So with this additional force, the first equation becomes:
\(T(OA) \sin \Delta - W(OC) \sin \theta - W_1 (OB) \sin \theta = 0\)
And since \(OA = AC = 1/5\; OB,\) we get:
\(T = \frac{(2W +5W_1) \sin \theta}{\sin \Delta}\)